Self-shrinkers for mean curvature flow
Mean curvature flow can be defined as the gradient flow of area which means that it deforms a given surface such that its area decreases the fastest. Surfaces which shrink homothetically under mean curvature flow without changing their shape are called self-shrinkers. They are of great importance for the general theory of mean curvature flow because they model local singularities developing along the flow.
References
- T. Ilmanen, Lectures on mean curvature flow and related equations, ETH Zürich (1998).
- R. Buzano, H. Nguyen and M. B. Schulz, Noncompact self-shrinkers for mean curvature flow with arbitrary genus, J. reine angew. Math. 818 (2025).
- D. Hoffman, F. Martin and B. White, Generating Shrinkers by Mean Curvature Flow, preprint (arXiv:2502.20340).
- D. Chopp, Computation of self-similar solutions for mean curvature flow, Experiment. Math. 3 (1994).
- D. Ketover, Self-shrinking Platonic solids, preprint (arXiv:1602.07271).
Noncompact self-shrinkers with one end
In his lecture notes on mean curvature flow, Ilmanen [1] conjectured the existence of noncompact self-shrinkers with arbitrary genus. Min-max techniques allow a rigorous existence proof for these surfaces [2]. Conjecturally, they have precisely one asymptotically conical end. For large genus this can be confirmed via a precise analysis of the limiting object of sequences of such self-shrinkers for which the genus tends to infinity.

(cross-section)
Noncompact self-shrinkers with two ends

(cross-section)

(cross-section)

(cross-section)

(cross-section)
Noncompact self-shrinkers with three ends

(cross-section)

(cross-section)
Compact self-shrinkers

(cross-section)

(cross-section)
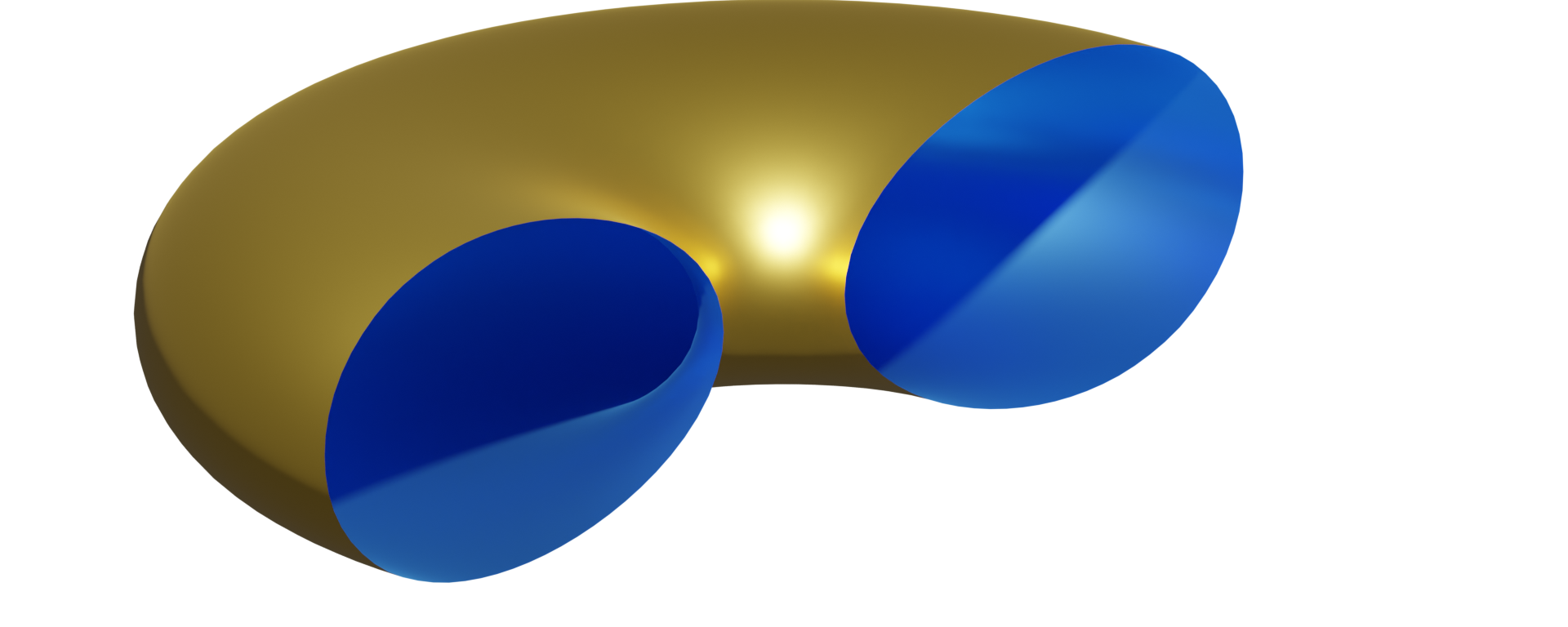
(cross-section)











